The Generalized Beta distribution provides a flexible way to specify a probability distribution A mathematical representation of the relative likelihood of a variable having certain specific values. It can be expressed as a PDF (or a PMF for discrete variables), CDF or CCDF. over a particular range (i.e., with a specified minimum and maximum). It is commonly used within project management simulations to represent the time to complete a task.
It is defined by specifying a Mean, Standard Deviation The square root of the variance of a distribution. The variance is the second moment of the distribution and reflects the amount of spread or dispersion in the distribution., Minimum and Maximum:
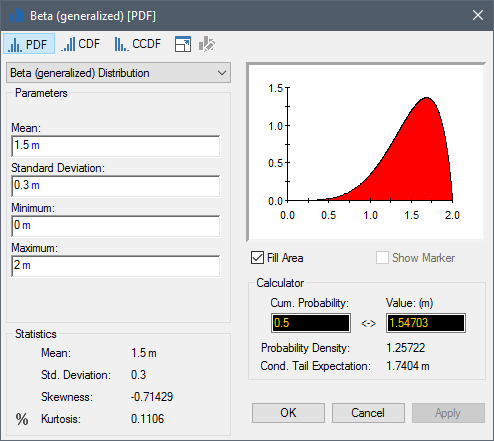
Note: The theoretical maximum standard deviation (SD) for a beta distribution (ranging from 0 to 1) is equal to sqrt[mean*(1-mean)]. However, with such a high SD, the beta distribution would have a “spike” of probability density at both its upper and lower bounds. With a somewhat lower SD, there will only be a spike at the limit closest to the mean. GoldSim restricts the SD to 0.6 of the maximum theoretical value in order to avoid having any spikes in the density function. If you need probability distributions that combine discrete spikes with continuous curves, you should construct this explicitly (by combining multiple distributions using an Expression, or by using a Cumulative distribution.
Learn more
- Beta Distribution
- BetaPERT Distribution
- Binomial Distribution
- Boolean Distribution
- Cumulative Distribution
- Discrete Distribution
- Exponential Distribution
- Externally-Defined Distribution
- Extreme Probability Distribution
- Extreme Value Distribution
- Gamma Distribution
- Generalized Beta Distribution
- Log-Normal Distribution
- Negative Binomial Distribution
- Normal Distribution
- Pareto Distribution
- Pearson Type III Distribution
- Poisson Distribution
- Sampled Results Distribution
- Student’s t Distribution
- Triangular Distribution
- Uniform Distribution
- Weibull Distribution